Effect
A spectator takes a deck of cards (they can examine it, the cards are regular and in no discernible order) and you ask them to remove either the Ace or Two of Hearts, and table it face down. Onto this, they are to place either the Three or Four of Hearts, and then finally, either the Five or Six of Hearts.
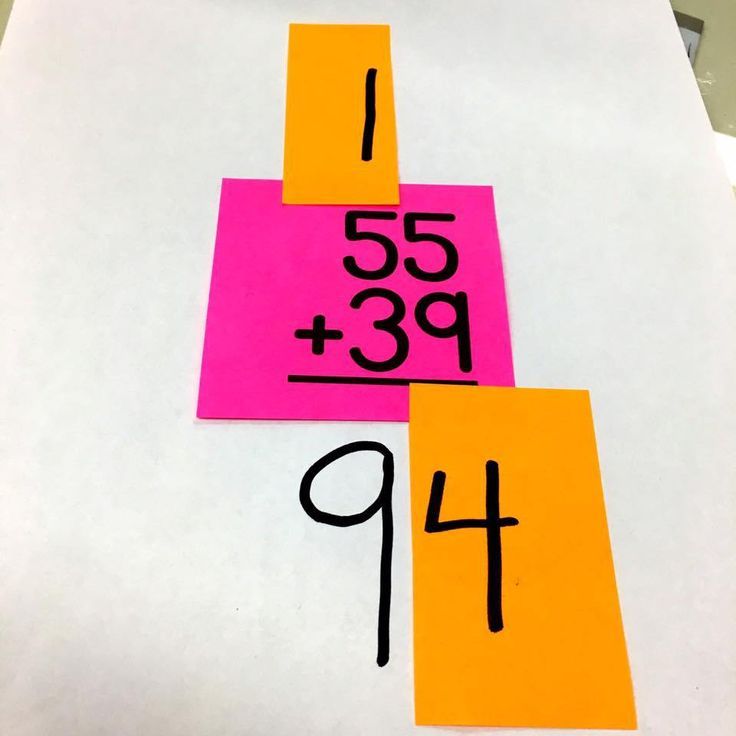
The deck is disposed of and the packet isolated between the spectators hands, in a pocket or the card case. With your best raised eye-brow, you impossibly reveal the total of the cards make 10.
The trick can be repeated for someone else, and the total may be different. The deck is regular, and the effect definitely borders on the 'nearly compelling' scale.
Discussion
So, the description above is a fair one, and a pretty bland example - it is possible to use as many pairs of cards as you like, giving a much wider range of totals. It is also possible to have three different spectators involved, each making one of the decisions, and so you can reveal the total before the spectators have turned over the cards themselves. And of course, once you know the number, it could be incorporated in any routine where that information could be advantageous, rather than simply blurted out.
Full credit for the effect goes to Bob Hummer, as this is a variation of his LITE-NING ADDITION from Half A Dozen Hummers. The method here is different, and interested parties should investigate Bob's solution for sure.
So, take your pack of cards, and remove the Ace to Six of Hearts. Separate them into odds and evens, and then mix each three card packet, so the order is random. Then, disperse all the odd Heart cards into the top third of the deck, and all the even Heart cards into the bottom third of the deck.
From here, it's simply a case of a simple false cut and/or shuffle, and then asking the spectator to think of either the Ace or Two of Hearts, and to remove it. Be sure to specify that they should keep the faces away from you at all times, and simply see what section of the pack the card is drawn from. This is repeated for the Three and Four, and then the Five and Six, and of course you are able to reveal not only the total, but also (if you wanted) the values of the individual cards.